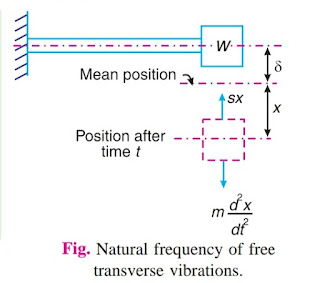
Consider a shaft of negligable mass whose one end is fixed and the other end carries a body of weoght W, as shown in fig.
Let s = Stiffness of shaft,
δ = Static deflection due to weight of the body,
x = Displacement of body from mean position after time t.
m = Mass of body = W/g As discussed in the previous article,
Restoring force = – s.x . . . (i)
and accelerating force = m d2 x . . . (ii)
dt2
Equating equations (i) and (ii), the equation of motion becomes
Hence, the time period and the natural frequntly of the transverse vibrations are same as that of longitudinal vibrations. Therefore
Note :
The shape of the curve, into which the vibrating shaft deflects, is identical with the static deflection curve of a cantiliver beam loaded at the end. It has been proved in the text book on strength of Materials that the static deflection of a cantiliver beam loaded at the free end is
δ = Wl3 (in metres)
3EI
Where W = Load at the free end, in newtons,
l = Length of the shaft or beam in metres,
E = Young’s modulus for the material of the shaft or beam in N/m2, and
I = Moment of inertia of the shaft or beam in m4.


No comments:
Post a Comment